Your Easiest imo problem images are available. Easiest imo problem are a topic that is being searched for and liked by netizens today. You can Find and Download the Easiest imo problem files here. Get all royalty-free vectors.
If you’re searching for easiest imo problem pictures information connected with to the easiest imo problem keyword, you have visit the right blog. Our site always gives you hints for viewing the highest quality video and picture content, please kindly hunt and find more enlightening video content and graphics that fit your interests.
Easiest Imo Problem. SOLVED IN ONLY TWO MINUTES. Most solutions to this problem first prove that f must be linear before determining all linear functions satisfying 1. Solved using Euclids algorithm. On each day students are given four and a half hours to solve three problems for a total of six problems.
What Are The Most Beautiful Imo International Mathematical Olympiad Problems Ever Quora From quora.com
This problem is considered to be one of the hardest problems ever because none of the members of the strongest teams ie. Substituting a 1b n gives fpfpn1qq fp2q2fpnq. IMO 2021 Problem 2. A sequence of real numbers a0a1a2is defined by the formula ai1 baichaii for i 0. The National Olympic Teams of the USA Russia or China succeeded to solve it correctly. Number Theory Level 3 d d d is a positive integer not equal to 2 5 2 5 2 5 or 13 13 1 3.
Let and be positive integers such that divides.
Solved using simple modulus. First note that if a0 0 then all ai 0For ai 1 we have in view of haii. The full IMO problem seems to be in addition to above. By design the first problem for each day problems 1 and 4 are meant to be the easiest the second problems. Endgroup ShreevatsaR Jul 19 11 at 1843 13 begingroup Answering the question mark in the title. Surely It was the legendry Problem 6 IMO 1988.
Source: medium.com
SOLVED IN ONLY TWO MINUTES. Let and be positive integers such that divides. The Hardest and Easiest IMO Problems The IMO is a two day contest in which students have 45 hours to solve three problems on each of the two days. You can view IMO problems on the official IMO website. IMO 1984 Problem 1.
Source: quora.com
The full IMO problem seems to be in addition to above. Solved using AM GM inequality. IMO 1964 Problem 1. Id like to discuss some of the problems given at this years International Mathematical Olympiad held virtually in St. A sequence of real numbers a0a1a2is defined by the formula ai1 baichaii for i 0.
Source: quora.com
The IMO competition lasts two days. Prove that fx 0 for all x le 0. The full IMO problem seems to be in addition to above. In particular fp0q 2fpn. Surely It was the legendry Problem 6 IMO 1988.
Source: quora.com
Solved using simple modulus. This cannot be the easy part because if you assume f00 then its easy to solve the rest of the problem. Videos you watch may. Here is a creative solution. If playback doesnt begin shortly try restarting your device.
Source: iq.opengenus.org
This problem is considered to be one of the hardest problems ever because none of the members of the strongest teams ie. Most solutions to this problem first prove that f must be linear before determining all linear functions satisfying 1. Endgroup ShreevatsaR Jul 19 11 at 1843 13 begingroup Answering the question mark in the title. Let and be positive integers such that divides. Solved using AM GM inequality.
Source: youtube.com
The first problem is usually the easiest on each day and the last problem the hardest though there have been many notable exceptions. Here is a creative solution. Here is a problem from the 2014 paper which is quite easy to understand though not that easy to answer. Surely It was the legendry Problem 6 IMO 1988. In particular fp0q 2fpn.
Source: pinterest.com
Most solutions to this problem first prove that f must be linear before determining all linear functions satisfying 1. Here is a creative solution. This cannot be the easy part because if you assume f00 then its easy to solve the rest of the problem. Let n² be an integer. A sequence of real numbers a0a1a2is defined by the formula ai1 baichaii for i 0.
Source: quora.com
Substituting a 0b n1 gives fpfpn1qq fp0q2fpn1q. The National Olympic Teams of the USA Russia or China succeeded to solve it correctly. IMO 1959 Problem 1. Today this problem seems laughably easy. Show that must be a perfect square Well Its seeming like a simple problem but it is nothing like that lets get some information about it.
Source: youtube.com
Id like to discuss some of the problems given at this years International Mathematical Olympiad held virtually in St. Number Theory Level 3 d d d is a positive integer not equal to 2 5 2 5 2 5 or 13 13 1 3. On each day students are given four and a half hours to solve three problems for a total of six problems. Today this problem seems laughably easy. Surely It was the legendry Problem 6 IMO 1988.
Source: youtube.com
PRMO RMO INMO It is a hard problem in first look but it actually becomes very easy to solve is we know and remember different properties of Circles Tang. An Easy IMO Problem. By design the first problem for each day problems 1 and 4 are meant to be the easiest the second problems. Today this problem seems laughably easy. SOLVED IN ONLY TWO MINUTES.
Source: quora.com
Show that must be a perfect square Well Its seeming like a simple problem but it is nothing like that lets get some information about it. Surely It was the legendry Problem 6 IMO 1988. Videos you watch may. About Press Copyright Contact us Creators Advertise Developers Terms Privacy Policy Safety How YouTube works Test new features Press Copyright Contact us Creators. An Easy IMO Problem.
Source: youtube.com
Substituting a 0b n1 gives fpfpn1qq fp0q2fpn1q. Nairi Sedrakyan is the author of one of the hardest problems ever proposed in the history of the International Mathematical Olympiad IMO 5th problem of 37th IMO. The full IMO problem seems to be in addition to above. Endgroup ShreevatsaR Jul 19 11 at 1843 13 begingroup Answering the question mark in the title. This cannot be the easy part because if you assume f00 then its easy to solve the rest of the problem.
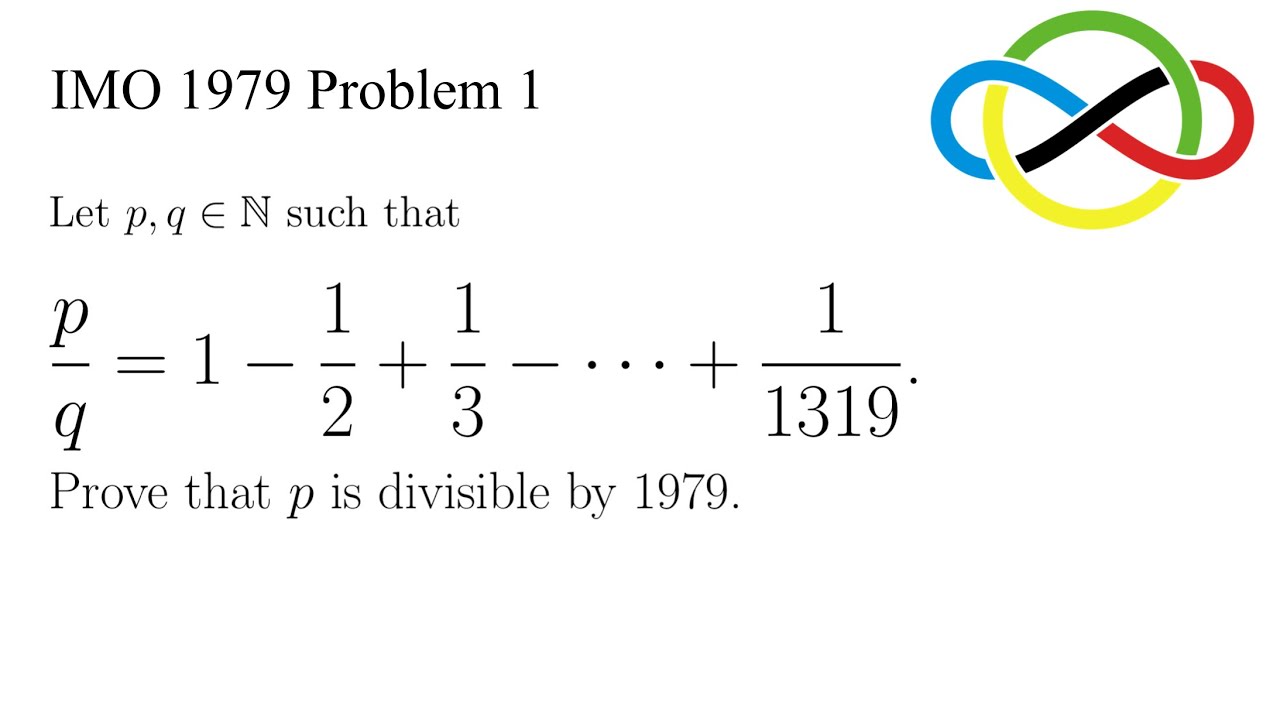Source: youtube.com
The National Olympic Teams of the USA Russia or China succeeded to solve it correctly. 63 rows Language versions of problems are not complete. An Easy IMO Problem. IMO 1986 Problem 1. To illustrate lets look at the very first problem of the very first IMO Problem 1 of 1959.
This site is an open community for users to share their favorite wallpapers on the internet, all images or pictures in this website are for personal wallpaper use only, it is stricly prohibited to use this wallpaper for commercial purposes, if you are the author and find this image is shared without your permission, please kindly raise a DMCA report to Us.
If you find this site good, please support us by sharing this posts to your preference social media accounts like Facebook, Instagram and so on or you can also bookmark this blog page with the title easiest imo problem by using Ctrl + D for devices a laptop with a Windows operating system or Command + D for laptops with an Apple operating system. If you use a smartphone, you can also use the drawer menu of the browser you are using. Whether it’s a Windows, Mac, iOS or Android operating system, you will still be able to bookmark this website.
